How to Integrate Common Core Math Practices into Content

Editor’s note: Leslie Texas and Tammy Jones are co-authors of the book series, Strategies for Common Core Mathematics: Implementing the Standards for Mathematical Practice, available from Routledge in three editions, K-5, 6-8 and 9-12.

by Leslie Texas and Tammy Jones

Middle school math teachers have a challenge—they need to help students become mathematical thinkers who truly understand concepts and don’t just memorize. One way we recommend doing this is by using the new Standards for Mathematical Practice (SMP) to help students build a foundation of thinking and communicating math. We find it most helpful to think of the standards as a door.

A door cannot function without a frame. The frame is the support system that holds the door in place. The two SMP that serve as the frame of the door are: (SMP #1) Make sense of problems and persevere in solving them; and (SMP #5) Use appropriate tools strategically.

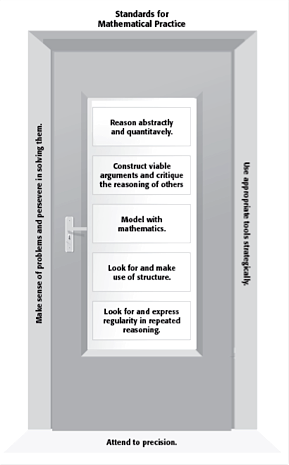
The threshold is another integral part of a door. The function of the threshold is to provide a transition between the inside and the outside or between rooms. (SMP #6) Attend to precision, serves as the threshold for the SMP doorway to the Common Core math standards. It is through precise and effective communication that students are able to gain insights about how they think about mathematics.

The remaining standards, (SMP #2) Reason abstractly and quantitatively; (SMP #3) Construct viable arguments and critique the reasoning of others; (SMP #4) Model with mathematics; (SMP #7) Look for and make use of structure; and (SMP #8) Look for and express regularity in repeated reasoning serve as the panels of the door.

The key to unlocking the door is to be deliberate and intentional in the implementation of these standard practices. It is one thing to say the SMP are embedded in daily instruction. It is another to actually seamlessly interweave the mathematical practices with content instruction.

The SMP in Middle School Math

The eight Standards for Mathematical Practice are not experienced in isolation. In fact, most of the time, students simultaneously employ several of the practices as they engage in mathematical experiences.

If students are to “construct viable arguments and critique the reasoning of others,” they will need to “attend to precision” by using precise vocabulary and symbolism. They will then check the reasonableness of their solutions by gathering supporting evidence. Students who “look for and make use of structure” will also “look for and express regularity in repeated reasoning” while they “make sense of problems and persevere in solving them.” Along the way they also “use appropriate tools strategically.”

Let’s look more closely at some of these Standards of Mathematical Practice — in the context of middle school:

1. Make sense of problems and persevere in solving them. Students look for a place to enter the problem and apply a strategy, then analyze to see if it is going to work or if they need to back up and start again. Middle school students must work with problems and tasks that require data to be collected and analyzed, and need to make predictions that connect to other disciplines.

2. Reason abstractly and quantitatively. Students move seamlessly between the verbal, the quantitative, and the symbolic representations of numbers and contextual problems. Middle school students should be using mathematics to solve problems in other contexts regularly: estimating reasonable answers, choosing efficient solutions, and identifying appropriate operations to use.

3. Construct viable arguments and critique the reasoning of others. Students listen to others explain their reasoning and critique it. Middle school students should be able to defend their work and give valid arguments to support what they did what they did. They should also be able to evaluate their peer’s work.

 4. Model with mathematics. Students take a contextual situation and translate it into a mathematical representation using math symbols and numbers to develop a deeper understanding of the mathematics that they are studying.

5. Use appropriate tools strategically. Students need to know and understand the benefits as well as the limitations of various tools. Middle school students should understand that tools are a way to explore and deepen their understanding of various concepts.

6. Attend to precision. Students who are mathematically proficient use clear and concise vocabulary, symbol notation, and gain deeper insights into the mathematics they are studying. Middle school students need to be encouraged to continue to write about and discuss mathematics using more mathematically precise language.

7. Look for and make use of structure. Students discover mathematical structures and patterns on their own. By middle school, numerical patterns can be expanded to discovering patterns and structures within algebraic expressions and the set of integers.

 8. Look for and express regularity in repeated reasoning. Students can recognize and build upon prior strategies to make connections between mathematical ideas. Middle school students should be given the opportunity to practice both deductive and inductive reasoning on a regular basis.

Strategies for Implementing the SMP

The following strategies will aid in unlocking, opening, and successfully going through the doorway of the Standards for Mathematical Practice to implement the CCSSM effectively.

♦ Problem-Solving Process (2 files) offers a graphic organizer and an approach for solving problems that can make contextual problem solving accessible to diverse students. The problem-solving process can be used to assist students in making sense of problems (Practice #1) as well as decontextualizing and contextualizing word problems (Practice #2). The process also requires students to construct viable arguments (Practice #3) as they formulate their own ideas about the meaning of the problem and make predictions about the outcome.

♦ Visual Vocabulary offers strategies for students to go beyond rote memorization of words to developing true understanding of mathematical terms, symbols, and notations. It employs forming concepts, comparing and contrasting concepts, and using a graphic organizer.

♦ Puzzling Problems offers a nonthreatening environment in which students can approach and engage in contextual problem solving while working cooperatively or independently.

♦ Grid Games and Matching Mania utilize games as a strategy for helping students gain content knowledge and understanding in a nonthreatening atmosphere. Students learn to work in cooperative group situations while practicing and strengthening their problem-solving skills.

These three activities are found in our book:

♦ The ABC Sum Race relies on reflective discussions and journaling. This activity involves students working in groups to solve problems through a competition. The ABC Sum Race provides an opportunity for students to “construct viable arguments and critique the reasoning of others.” Students work both collaboratively and individually while solving problems. Assigning students a specific letter and providing leveled problems makes the activity easily differentiable. The problems can be word problems, procedural problems, or computational problems, as desired.

♦ Walk This Way utilizes simulations and role-playing to help students develop understanding of concepts. Kinesthetic learning occurs by direct experience, and learning transpires as a result of what was done rather than what was said or read. Through this strategy students build multiple representations and use repeated reasoning to investigate mathematical concepts on horizontal and/or vertical number lines as well as the Cartesian coordinate plane by physically representing mathematical situations on a life-size graph.

♦ What’s My Move? uses reflective discussions and probing questioning to develop a deeper understanding of the content being studied. Students are engaged in a task that requires them to reason to find solutions to the problem posed. They have to justify their findings while using precise mathematical language, symbols, and notation. The students while investigating this task can employ a variety of tools. Through discerning various possible patterns, students are asked to develop a mathematical model to describe the physical movements of the participants.

_______

Collectively, Leslie Texas and Tammy Jones have almost 40 years of classroom experience in teaching in elementary, middle, and high school.

Tammy served as a writer and the content editor for the Tennessee Mathematics Curriculum Framework and the Tennessee Mathematics User’s Guide. She also served on the Mathematics Feedback Group for the Common Core Standards. 

Leslie has taught middle and high school mathematics and science, and has strong content expertise in both areas, including a degree in mathematics and physics. Leslie earned her M.Ed. from the University of Louisville, with an emphasis in interdisciplinary curriculum, and is working on her doctorate.

In their math consulting businesses, Leslie and Tammy work directly with classroom teachers, modeling and offering continued support throughout the year to build capacity at the building and district levels.