Use Kids’ Questions to Launch Math Adventures
By Jerry Burkhart
In a recent MiddleWeb post, I shared examples of Creative Math Prompts, a type of inquiry-based math task that cultivates learners’ curiosity, creativity, and independent reasoning skills. In this article, I want to share some of my experiences in using a Creative Math Prompt with students to encourage them as “adventurous learners.’
We began with the subtraction expressions below. As a math educator, what do you notice? What questions might you ask?
As you read on, I hope you’ll continue to ask questions and explore along with us. The possibilities are endless!
Getting started
This school year, I have been meeting weekly with 25 fourth-graders to extend their learning from math class. The prompt above was our first activity. I wanted the students to gain a richer, more flexible understanding of place value, subtraction, and multiples. I also hoped to get them asking as well as answering questions. During our first meeting, we discussed what it means to be a “math adventurer.”
Math adventurers are curious.
Math adventurers take risks.
Math adventurers are flexible thinkers.
We recognized that curiosity, courage, and flexibility are important traits for all types of adventurers. Our discussion set the stage for continued conversations throughout the year.
After distributing copies of the subtraction expressions, I asked students to write at least one or two things that they noticed and wondered. This was a new experience for them, and we didn’t rush it.
I reassured them that there were no right or wrong answers and that it was okay, even helpful, to include ideas that seemed simple or obvious. Here are a few of their responses:
Look at the wonderful mix of ideas and questions! One comment (“This isn’t that hard”) showed me that some students, expecting a certain type of challenge, may have been surprised at doing familiar subtraction computations. Other students were looking for meaning and relevance. (“Why are the numbers backwards?” “Why are we doing this?”).
Many comments described what students saw on the page. (“Every equation is the number at the beginning flipped around.” “They are all 3-digit numbers.”) Others dealt with what they noticed after subtracting. (“All of the differences are 3-digit numbers.” “The answers all have 9 in the tens.” “The first and last digits in the answers add up to 9.”) I was pleasantly surprised that students used place value language so often and effectively.
Beginning to explore
All in all, I felt excited and a little overwhelmed with the number of ideas. I decided to begin by asking students to look through the list of “I notice” statements and say whether they agreed or disagreed and why. Students expressed doubts about some of them. Other statements needed clarification. For example, “The top ones are all crossed off” came from a student who noticed that he needed to regroup/”borrow” constantly. It was a perfect chance to suggest trying a variety of algorithms!
Soon we were ready to focus on the “I wonder” statements, but we had a hard time getting started—partly because students had asked so many challenging “Why?” questions! I began to feel that we needed to explore more examples of equations first, so I decided to step in and show them a list of questions that mathematicians often ask: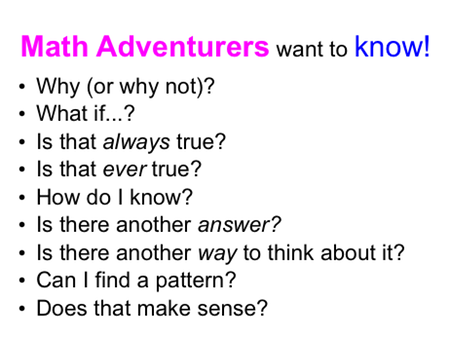
After acknowledging their great “Why?” questions, I suggested that we try to come up with an “Is that always true?” type of question. My hope was to lead them toward creating more equations, but it didn’t happen. To them, always meant “every expression on the page” rather than “every expression like these.” They were not imagining beyond what they saw on their handouts. I was glad to be at end of our session in order to have more time to plan the next step!
Gathering and organizing the data
While preparing, I decided to create a name for “subtracting a number written backwards.” I called it backtraction. (Students liked the name, but I wish now I had let them invent their own.)
I hoped it would help them think “beyond the page” if I asked them to create their own “three-digit backtraction” expressions. I encouraged them to try alternatives to the traditional paper-pencil algorithm for some of their calculations.
This idea worked better. Students became very enthusiastic, and we now had new equations to look at. They noticed that many of their observations about the differences remained true. “9” kept showing up as the tens digit, and the hundreds and ones digits had sums of 9. For example:
However, we also saw some exceptions, and we were getting so many equations that it was hard to keep track of them. I suggested that we organize our data. We put one equation each on a post-it note and stuck it to a board containing other equations having the same answer. This picture shows part of what we had after about 20 minutes (click to enlarge).
Though you probably can’t read the equations, notice that each board has an answer at the top and that some boards have very few post-it notes. When we double-checked the calculations on these boards, we discovered errors. After we corrected them, all of our answers fit the patterns we had noticed earlier—with one exception: we had a board for “0.”
New discoveries
We were making progress, but our data were still disorganized. One student suggested arranging the boards in numerical order.
0, 99, 198, 297, 396, 495, 693, 792
Students now saw some new patterns. The hundreds digits were increasing by 1, and the ones digits were decreasing by 1. From this, they predicted that the list should also include 594, 891, and 990. We searched for backtraction calculations having these answers. Equations for 594 came pretty quickly (example: 862 – 268 = 594). 891 was harder, and we never found one for 990. Eventually, when I prompted students to focus on the numbers instead of the digits, they recognized that all of the answers (including 0) were multiples of 99!
Students were now better prepared to go back to their earlier questions and to develop new ones. All in all, we spent about ten class sessions on this investigation. I was surprised and impressed by the students’ ability and willingness to stick with one project for so long!
The process felt messy but exciting. I struggled with some things along the way, especially balancing students’ questions and ideas with my desire to move their thinking in certain directions.
It will be easier next time, because I have a clearer understanding of how they think about the ideas. I might even try having students formally present some of their questions, discoveries, and reasoning to others.
Learning more about our experiences
Read here to learn more about our exploration. This page at my site also includes ideas for using the prompt with older and younger students.
Upon reflection, I feel that my students and I made many exciting discoveries, yet we barely scratched the surface of the possibilities. I would love to continue the investigation next year with the same group as fifth graders! I hope that some of you will try it with your students and share your experiences on this forum or by contacting me.
Further resources
Creative Math Prompts by Jerry Burkhart
Advanced Common Core Math Explorations book series, by Jerry Burkhart.
Extending the Challenge in Mathematics, by Linda Jensen Sheffield. Corwin, 2003.
Intrepid Math, by Jerry Burkhart
Projects That Never End, by Jerry Burkhart
_________________________
Jerry Burkhart is a mathematics educator and consultant with over 30 years of classroom experience. He is the author of the book series, Advanced Common Core Math Explorations, published by Routledge/Prufrock Press, and he presents regularly at state, regional, and national conferences on topics related to mathematical talent development.
As founder of 5280 Math Education, he helps schools and districts implement research-based programming for gifted math students and offers tools and strategies for developing and nurturing adventurous math learners. Visit his store at MyEdExpert.
